We use Archimides' principle, which states that the buoyant force on an object is always directed upward and its magnitude is equal to the weight of the fluid displaced. Recall that a fluid can be either a gas or a liquid. So, let
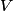 denote that volume of the balloon. We neglect the volume of the suspended mass. The buoyant force is then denote that volume of the balloon. We neglect the volume of the suspended mass. The buoyant force is then  . This must equal the weight of the helium-filled balloon plus the weight of the . This must equal the weight of the helium-filled balloon plus the weight of the  mass. That is mass. That is This can be easily solved for 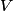 : :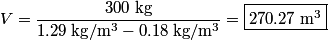 Therefore, answer (D) is correct. |